

Organize all the necessary information into a table, then total the moments of inertia of the parts to get the moment of inertia of the whole shape. on Lorentzian circle (its total rotation angle is ) with the polar moment of inertia T. When solving for the moment of inertia of a composite area, divide the composite area into basic geometric elements (rectangle, circle, triangle, etc) for which the moments of inertia are known. Fulltext - On Polar Moments of Inertia of Lorentzian Circles. 5.11 Consider a set of orthogonal axes inclined at 45.
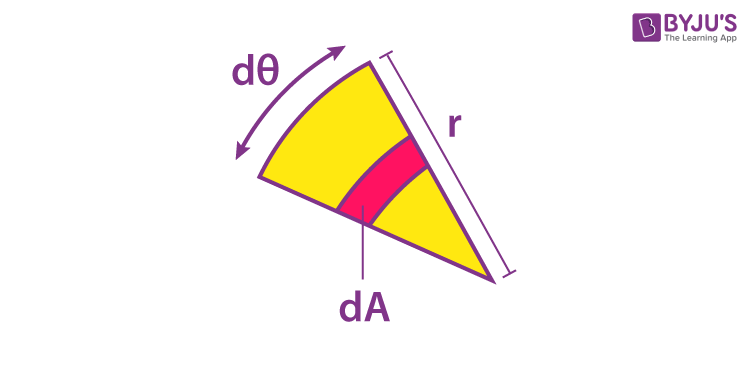
In some cases, there is a possibility to calculate the M.O.I of the circle regarding its axis tangent to the perimeter, then we will practice the parallel axis theorem.\(\require Solution 2.Identify and divide the complex shape into basic shapes for easier computation of moment of inertia. Using Mohr's circle, determine the moments and product on inertia with respect to this set of axes. from the requirement that the two moments of inertia Iyy and Izz assume extremal values, i.e. Let us just put the value in the integral. A representation of the inertia circle is given in Fig. Let us just simplify this integration with proper steps:Īs we know about the trigonometric identity i.e. The calculation for the moment of inertia tells you how much force you need to speed up. A differential element of area in the form of a circular ring may be used for the calculation of the moment of inertia about the polar z-axis through O. Now, let us just transcribe the integral for the moment of inertia of a circle. Slivers under the curve are green (click the circle below). Now after the differentiation, the expression will be obtained as Presently, let us just describe the coordinates by applying the polar system.Īfter the completion of step-1, we need to calculate the differential area, which can be achieved by declaring the area of the sector.įrom the image, the area of the sector can be expressed as

I y is the component of MOI in the y-axis. Moment of inertia about the x-axis: displaystyle Ix int y2, dA. Let us just begin by remembering the equation for the second-moment area. This explanation will follow certain steps, such as: Obviously, if you want the polar moment of inertia, you should not. Expressed in diameter of the circle D, the above equation is equivalent to: UnitsThe equations listed for the moment of inertia of the circle reveal that. The moment of inertia of the semicircle is generally expressed as I r 4 / 4. is just computing the polar moment of inertia and dividing by two. As a guess the second term, NIb, can be ignored for most cases. d is the perpendicuar distance between the centroidal axis and the parallel axis. The moment of inertia formula of a circle, as per the derivation, the circular cross-section will be calculated with the radius and an axis going exactly through the center. d diameter of pattern, Ib polar moment of inertia of one bolt.
#Moment of inertia of a circle how to
It will be easier to understand how to find moment of inertia of a circle with this derivation. This expression for the moment of inertia of a circle about its diameter can be given asĭo you know how to find the moment of inertia of a circle? To learn about this, we need to understand the derivation of the moment of inertia of a circle, as explained below. If we consider the diameter of a circle D, then we must also take ‘r’ the radius as D/2. Moment of Inertia of a Circle about its Diameter
In the case of a quarter circle the expression is given as: In case of a semi-circle the formula is expressed as: In the case of a circle, the polar moment of inertia is given as: Similarly, the moment of inertia of a circle about an axis tangent to the perimeter(circumference) is denoted as: The moment of a circle area or the moment of inertia of a circle is frequently governed by applying the given equation: The moment of Inertia formula can be coined as: Mathematically, it is the sum of the product of the mass of each particle in the body with the square of its length from the axis of rotation. But in this case the mass of half of the circle is M so 2 M will be the mass of the whole circle. Yes, the proper definition of the moment of inertia is that a body tends to fight the angular acceleration. We know that the moment of inertia of a whole circle with mass M is I 2 1 M R 2. Think of a solid disk and a hoop rolling down a ramp. When a body starts to move in rotational motion about a constant axis, every element in the body travels in a loop with linear velocity, which signifies, every particle travels with angular acceleration. An objects moment of inertia is a measure of its resistance to being rotated about an axis. It can be inferred that inertia is related to the mass of a body. First of all, let us discuss the basic concept of moment of inertia, in simple terms.


 0 kommentar(er)
0 kommentar(er)
